Chapter 1: Introduction to Orbital Mechanics

In my previous article published on February 1st, I began a journey into the complexities of space, and this piece continues that exploration.

When navigating an airplane, ascending involves simply tilting the nose upward. If conditions allow, the aircraft will rise. The same principle applies to lateral movement; a left turn will direct you left—typically. However, the principles governing orbital mechanics defy such straightforward logic and can lead to significant confusion.

To ascend in orbit, one must increase speed. To shift laterally? There’s no distinct “right”—everything is relative. This concept is often the most challenging for me to comprehend: the absence of “right” or “left.” We tend to impose our terrestrial navigation methods onto orbital paths, which can result in misunderstandings. Consequently, new terminology has emerged, sharing a resemblance with familiar terms but carrying subtly different meanings.

The terminology includes six primary directions of movement, which do not relate to our orientation but instead to our trajectory. This distinction is crucial in understanding orbital navigation. Most cultures might say, “move right” or “ascend,” while some—like those in military contexts or Southern California—may use cardinal directions. A few cultures avoid personal reference points entirely, referring to movement simply as “go north” or “go east,” demonstrating a unique approach to navigation.

Section 1.1: Key Directions in Orbital Mechanics

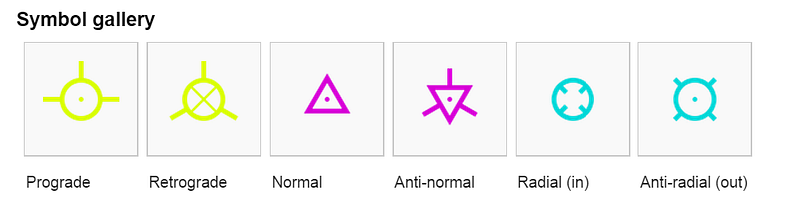
In an orbital context, the terms PROGRADE and RETROGRADE come into play. In orbit, motion is constant; if you're not moving, you're merely falling. Prograde is the direction of your movement, while retrograde is the opposite. The concept of “going faster” in an orbit diverges from typical understanding—accelerating in one part of the orbit may mean slowing down elsewhere, resulting in an extended orbital period.

The concepts of NORMAL and ANTI-NORMAL relate to altering the inclination of your orbit in relation to the equator of the celestial body you’re orbiting. For instance, an inclination of zero places you directly above the equator, while 90 degrees denotes a trajectory that crosses from one pole to the other. If you launch from a latitude such as Cape Canaveral (28°N) and head directly east, your orbit will share this inclination.

Section 1.2: Maneuvering in Orbit

To adjust your inclination, you would orient your rocket either NORMAL or ANTI-NORMAL and engage your engines until the desired inclination is achieved. This adjustment is most efficient at the APOGEE, the highest point of your orbit, while PERIGEE marks the lowest. All orbits are elliptical, and perfect circularity is a rarity.

The final two directional terms, RADIAL IN and RADIAL OUT, are less commonly utilized for maneuvers since prograde or retrograde burns at different orbital points tend to be more efficient. Radial-in directs your rocket toward the center of the body you’re orbiting, while radial-out does the opposite.

One might assume that orienting toward the planet would decrease altitude, but orbital mechanics often contradicts intuition. Initially, you may descend, but as long as you avoid atmospheric drag or surface impact, you will ascend higher on the opposite side of your orbit.

Chapter 2: The Challenges of Rendezvous in Space

In the first video, "The Only Video Needed to Understand Orbital Mechanics," viewers are provided with essential insights into the fundamental principles that govern movement in space.

To successfully rendezvous with another spacecraft, it’s crucial to first align inclinations. This initial step is non-negotiable, as differing velocities will hinder any attempt at coordination. After matching inclinations through normal and anti-normal burns, we can proceed to synchronize speeds.

Assuming both orbits are circular simplifies the process. If our target orbits at 400 km while we sit at 600 km, we must acknowledge that the lower orbit moves at a quicker pace. To align with our target, we must decelerate. By performing a retrograde burn, we temporarily reduce speed, which also lowers our perigee, enabling us to gain speed overall.

However, we cannot simply decelerate at random intervals. Timing is critical; we need to adjust our perigee to match the target's altitude of 400 km. Once at our apogee, we decelerate to sync our orbits perfectly with the target's trajectory.

After half an orbit, as the target gradually approaches, we realize that despite being at the same altitude, our speeds differ because our apogee is higher than that of the target. We must decelerate once more to equalize our velocities. Subsequently, with our speeds aligned, we can easily navigate closer using smaller thrusters.

In conclusion, this exploration into orbital mechanics reveals the intricacies involved in space navigation. I hope you found this discussion enlightening. Part three will follow soon, and I welcome any comments or questions you might have. Engaging with readers is invaluable as I navigate this complex landscape of science and technology.

The second video, "Orbital Mechanics - Hill's Equations and Spacecraft Formation Design," delves deeper into advanced concepts in orbital mechanics, enhancing our understanding of spacecraft dynamics and formation strategies.