Engaging with Geometry: A Fun and Simple Challenge
Written on
Chapter 1: Introduction to the Geometry Puzzle
In this section, we will explore an intriguing math challenge centered around Thanom's roll of paper. Could it be that he has caused some chaos in the kitchen? To assist you, here's a helpful tip: creating a diagram will be beneficial.
I encourage you to take a moment to pause, grab some paper and a pen, and attempt to solve this puzzle on your own. Once you're ready, continue reading for the solution!
Section 1.1: Understanding the Problem
We know that the diameter of the roll is 12 units, while the diameter of the inner tube measures 4 units. This gives us radii of 6 and 2 units, respectively. The area of the inner tube is calculated as (2^2pi = 4pi). This detail will be significant as we proceed.
To visualize the problem, here’s a diagram for your reference.
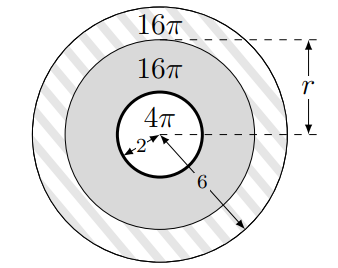
Section 1.2: Finding the Remaining Roll's Dimensions
Our objective is to determine the diameter of the remaining portion of the roll, which we have denoted as having a radius (r). Once we establish (r), we can easily find the diameter by simply doubling that value.
From a top-down view, the area of the entire roll can be represented as follows:
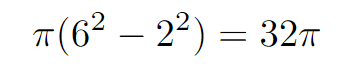
After utilizing half of this area, the remaining portion of the roll can be depicted as:
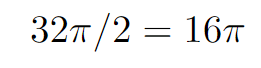
Including the area of the inner tube, which we know to be (4pi), the total area of the complete circle must equal (20pi).
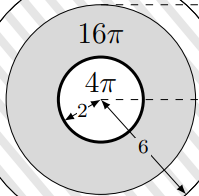
With this information, we can derive the radius of the remaining roll:
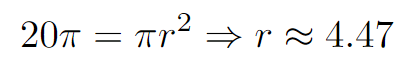
Consequently, the diameter is simply twice the radius, resulting in approximately 9 cm. Thus, (D) is the solution to our puzzle.
What were your thoughts while working through this? Feel free to share in the comments; I'm excited to hear your insights!
Section 1.3: Conclusion and Further Exploration
If you enjoyed this puzzle, I invite you to explore more math challenges on Medium, featuring a variety of topics like Algebra, Geometry, and Calculus.
Your engagement means a lot! If you found this article valuable, please consider clapping for it.

If you're feeling generous and wish to support my work, consider buying me a coffee. It helps immensely with my writing journey and personal endeavors.
Happy Solving!